Use of calculator

Comparison is made to find out which fraction is greater and which is smaller. Mixed numbers and improper fractions are used to compare with is calculator.

Ways to compare fractions

·         Change the mixed numbers to improper fractions.

·         Find out the LCD for the fractions.

·         Must change each fraction into equivalent with least common denominator

·         Make comparison: you can compare the numerator if the denominators are similar. Fraction With a greater numerator is the larger.

For example:

5/6 and 3/8 Compare.

Find out the Least common denominator: multiples of 6 are 6, 12,18,24,30, etc. Where the multiples of 8 are 8,16,24,32, etc. As such the lowest common multiple is 24 so we take LCD. Using LCD change each fraction to its equivalent fractions. For 5/6 multiple n and d by 4 to have LCD = 24 in the denominator.

    5/6 * 4/4 = 20/24

For 3/8, multiply n and d by 3 to get LCD =24 in the denominator.

    3/8 * 3/3 = 9/24

Now compare the fractions. As such there are like denominators so you can make comparison with numerators.

  Hence 20/24 > 9 we conclude 5/6 > 3/8

For further help see the fraction calculator to understand it.

You can use this calculator to add, subtract, multiply or divide the fractions. Answer to the questions are provided as simplified fractions in lowest terms or as mixed numbers in minimize form. Input proper and improper fractions, then select the mathematics operations, proceed and click calculate.

You can add up, subtract, multiply or divide negative fractions. Place a negative or minus sign before the numerator or the top number. Example if one of your fractions is -16/20 then just place minus sign before the “16” and now proceed to enter all other n and d.

Choose the mathematics operation and click calculate.

To make calculation with whole numbers and fractions use mixed numbers calculator.

The mixed number calculator can add, subtract, multiply, and divide whole numbers of fractions.

Do mathematics calculations with whole numbers or mixed fractions and perform operations on fractions.

Put the whole number or mixed numbers in the following ways:

·         The whole numbers up to 3 digits in length.

·         Now enter as ¾ which is a three fourth. You can also enter up to 3 digits in length for each n and d. For example: (456 / 789)

Equations

For adding fractions the algebraic formula is:

 for example:               

2/6 + ¼ = (2 * 4) +(6 * 1) / 6 * 4 = 14/24 =7/12

Subtracting fractions

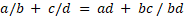
      For example:         

        2/6 – ¼ = (2 * 4) – (6 *1) / 6 *4

            =2/24 = 1/12

Multiplying the fractions

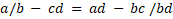
For example:

2/6 * ¼ = 2 * 1 / 6 * 4

= 2/24 =1/12

Dividing of fractions

The basic formula for dividing fractions is:

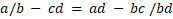
     Steps: 2/6 ÷ ¼ = 2 * 4 /6 * 1

     = 8/6 = 4/3 = 1+1/3