Using the Equation to Find the Intercepts

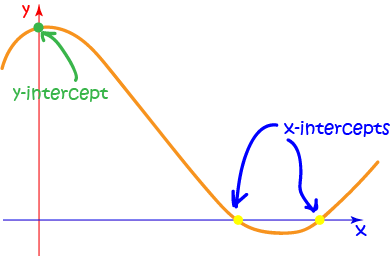
What are Intercepts?

Intercepts are places where the graph of a function cuts the axes. As there are two axes in the \(xy\)-plane, there are two different types of intercepts:

• \(x\)-intercepts occur when the graph crosses the \(x\)-axis.
• \(y\)-intercepts occur when the graph crosses the \(y\)-axis.

Finding the Intercepts

The \(x\)-intercepts lie on the \(x\)-axis, so their coordinates have the form \((x,0)\).

The \(y\)-intercepts lie on the \(y\)-axis, so their coordinates have the form \((0,y)\).

Examples

Example 1

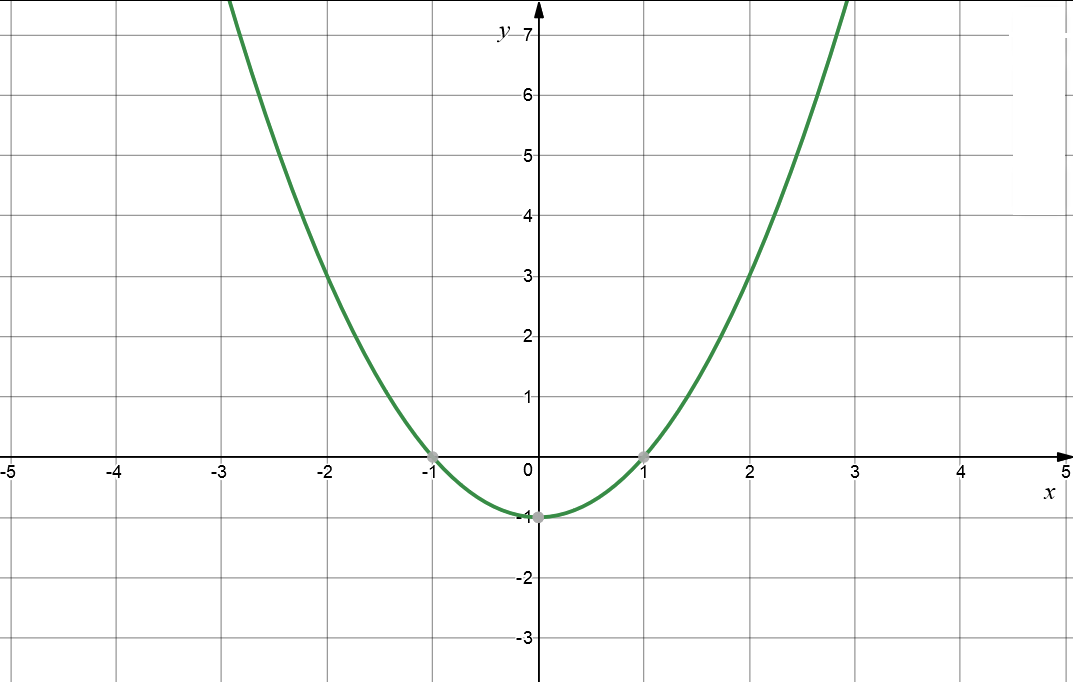
Find the intercepts of the function \(y= x^2 - 1\).

Solution:

The \(x\)-intercepts occur when y = 0:

The \(x\)-intercepts are at \((1,0)\) and \((-1.0)\).

The \(y\)-intercepts occur when x = 0:

The \(y\)-intercept is at \((0,-1)\) .

The graph looks like:

Example 2

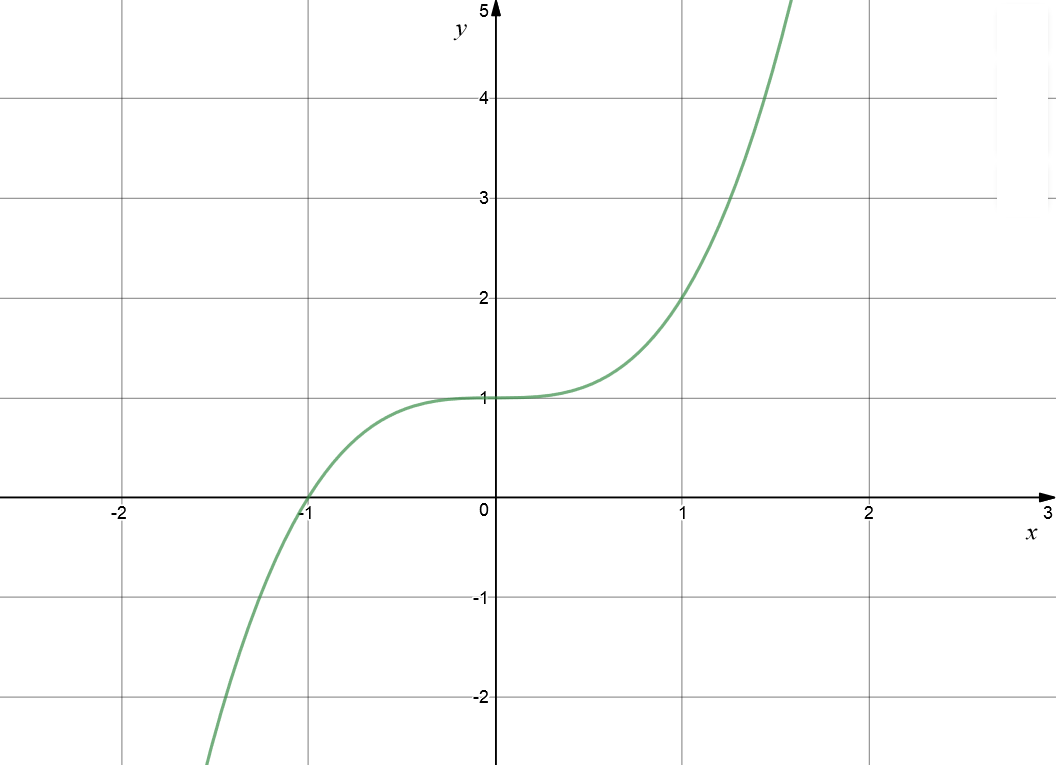
Find the intercepts of the function \(y= x^3 + 1\).

Solution:

The \(x\)-intercepts occur when y = 0:

The \(x\)-intercept is at \((-1.0)\).

The \(y\)-intercept occurs when x = 0:

The \(y\)-intercept is at \((0,-1)\) .

The graph looks like:

Example 3

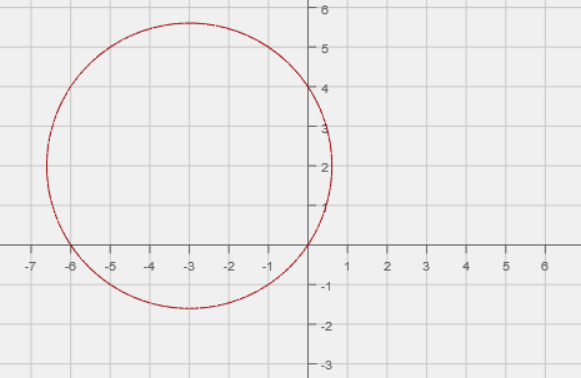
Find the intercepts of the function \( x^2 + 6x + y^2 - 4y = 0 \).

Solution:

The \(x\)-intercepts occur when y = 0:

The \(x\)-intercepts are at \((0,0)\) and \((-6,0)\).

The \(y\)-intercepts occur when x = 0:

The \(y\)-intercepts are at \((0,0)\) and \((0,4)\).

The graph looks like: