Math Definitions - Letter C
Chapters
Complex Number
Definition of Complex Number
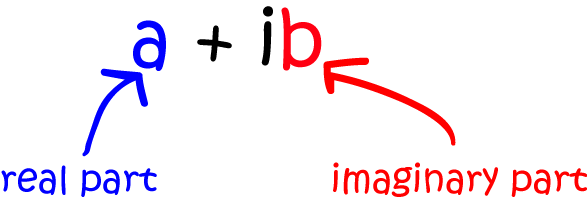
The complex numbers were introduced to provide a number system in which every number (positive or negative) has a square root.
The introduction of the imaginary number \(i = \sqrt{-1}\) accomplished this purpose.
Each complex number can be written in the form \(a + ib\), where \(a\) and \(b\) are real numbers, which can be zero.
We call \(a\) the real part of \(a + ib\), and \(b\) the imaginary part of \(a + ib\).
All real numbers are complex numbers.
Some examples of complex numbers are \(1 - i\), \(2 + 3i\), \(-3.5i\) and \(17\).
Description
The aim of this dictionary is to provide definitions to common mathematical terms. Students learn a new math skill every week at school, sometimes just before they start a new skill, if they want to look at what a specific term means, this is where this dictionary will become handy and a go-to guide for a student.
Audience
Year 1 to Year 12 students
Learning Objectives
Learn common math terms starting with letter C
Author: Subject Coach
Added on: 6th Feb 2018
You must be logged in as Student to ask a Question.
None just yet!